Researchers from the University of Ottawa (uOttawa), in collaboration with the Weizmann Institute of Science and Lancaster University, have observed a hidden quantum transition that can only be seen depending on how observers perform measurements.
An essential part of the scientific method relies on the ability to measure the output of an experiment accurately and to juxtapose these findings with previous results. Scientists develop measurement devices, or meters, which enable them to precisely quantify the magnitude of physical properties. However, the ‘measurement process’ raises a critical and intriguing question: does the process of measuring a parameter alter the system being measured? In physics, it is often believed that the influence on the system is negligible. Yet, the same assumption cannot be made in the context of quantum mechanics, as the act of measurement can significantly impact the system under observation.
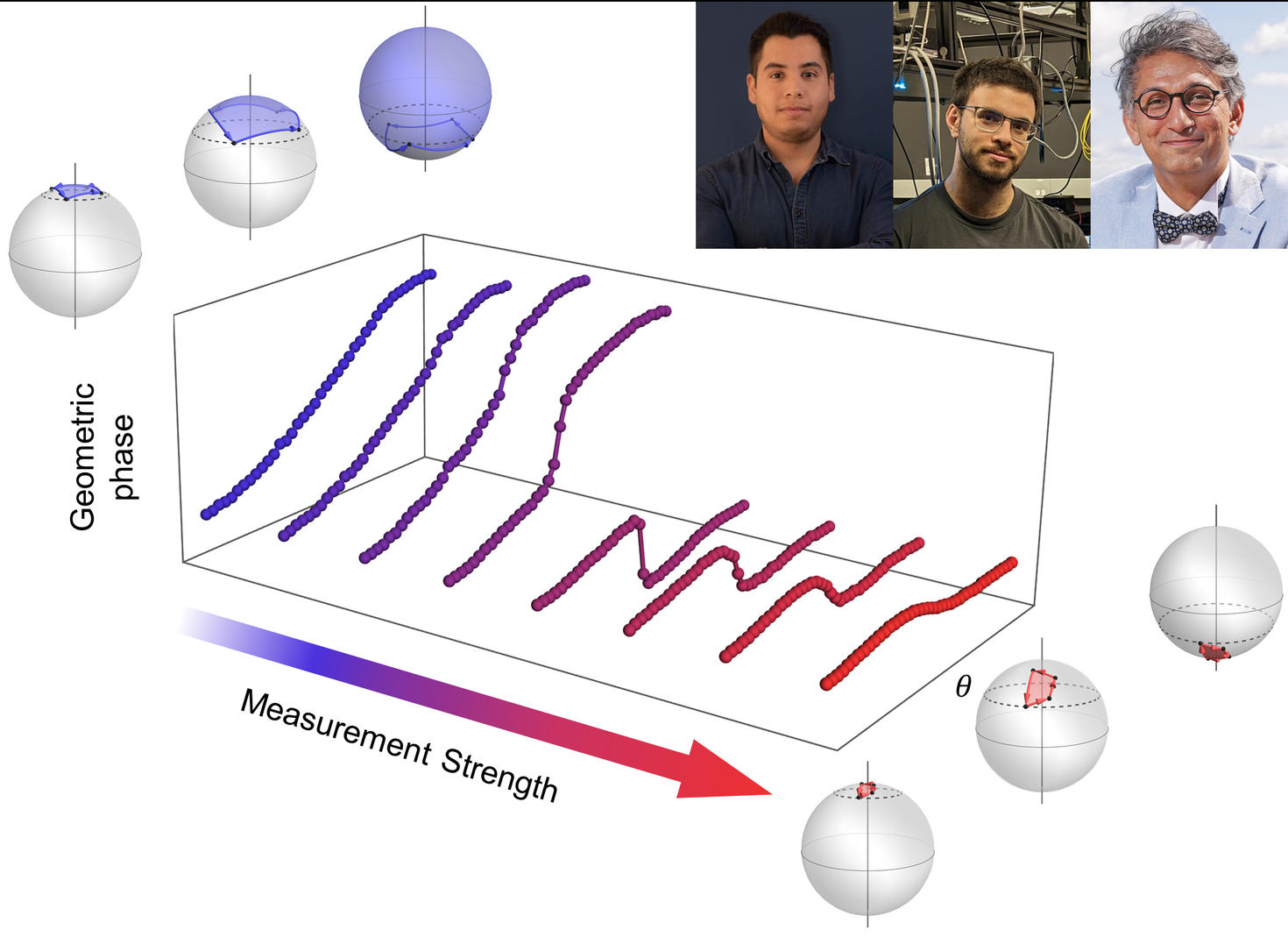
The research team, led by Yuval Gefen, professor in the Faculty of Physics at the Weizmann Institute of Science and Ebrahim Karimi, professor of physics and Canada Research Chair in Structured Quantum Waves at the Faculty of Science at the University of Ottawa, devised a sophisticated protocol to observe this measurement-driven topological transition. This protocol entailed a cyclic sequence of measurements with varying strengths—from strong to weak—on the polarization state of photons emitted from a laser source. Their findings reveal that while the topological transition remains intact despite the presence of imperfections in both the system and the process, it is also sensitive to these imperfections.
“This sensitivity manifests in significant alterations in the transition’s location and shape, underscoring the delicate balance between system integrity and external influences in such advanced scientific explorations,” said Manuel F. Ferrer-Garcia, Ph.D. candidate, who conducted the lab experiment at the Nexus for Quantum Technologies Institute at uOttawa.
In quantum mechanics, it is widely accepted that the entirety of a quantum system’s state is encapsulated within its wave function. To learn about the state, the system interacts with a measuring device, i.e. meters, which are instrumental in quantifying the magnitude of a physical property. Conventionally, quantum scientists employ a technique known as projective measurements in their laboratory. These measurements are considered strong since they provoke the “collapsing” of the wave function, where it is reduced to a specific state aligned with one of the measuring device’s states. This process not only yields information but also alters the initial quantum state of the system. However, it is feasible to devise a measurement protocol that minimally influences the system, leading to somewhat indeterminate readings on our meter. Through repeated interactions, it becomes possible to gather information about the system, a process termed weak measurements. From this understanding, we can deduce the potential for designing measurement protocols whose impact ranges between these two extremes—strong and weak. This concept opens up new avenues for exploring quantum systems and their interactions with measuring devices, marking a significant stride in quantum measurement techniques.
A significant yet less apparent aspect of quantum phenomena is their deep connection with topological concepts. Topology, in essence, is a branch of mathematics focusing on the study of properties that are invariant or change discontinuously under continuous deformations. An example of these invariants is the number of holes in closed surfaces – e.g., a sphere can be continuously transformed into a doughnut shape, but the number of holes will abruptly change from zero to one when two different surface points come into contact with each other. Topological invariants play an important role in many areas of modern physics. In this work, the researchers observed a topological transition when the measurement strength changes from strong to weak. This transition involved the behaviour of another mathematical concept: the geometric or Pancharatnam-Berry phase. When a quantum state undergoes a cyclic evolution, i.e. it goes back to the initial state after a period of time, it can acquire a “global” phase, which is due solely to the curvature of the space where the evolution happens. This phase can be observed by interfering with the evolved state with the initial one.
The impact of this research goes beyond the extent of fundamental physics. Since the transition proved to be sensitive to certain features of the quantum system, it has the potential to be used in sensing applications or characterization of optical elements.
The study ‘Topological Transitions of the generalized Pancharatnam-Berry phase’ was published in Science Advances on 24 November 2023.
